This is an excerpt from the book series Philosophy for Heroes: Continuum.
Is light a wave or a particle?
[…] when we allow for the dynamics of gravity and quantum mechanics, we find that [our] commonsense notion is no longer true. This is the beauty of science, and it should not be threatening. Science simply forces us to revise what is sensible to accommodate the universe, rather than vice versa. —Lawrence Krauss, A Universe From Nothing [Krauss, 2012, p. 151]
Quantum mechanics could just as well be called “wave mechanics” [cf. Bell, 1988, p. 187]. That is to stress the fact that classical mechanics dealt with the universe as comprised of particles as if they were small billiard balls, while quantum mechanics sees reality as particles acting as waves, not as billiard balls. Once we group particles together as larger, visible objects, the results from classical “billiard” mechanics are good enough to explain most phenomena.
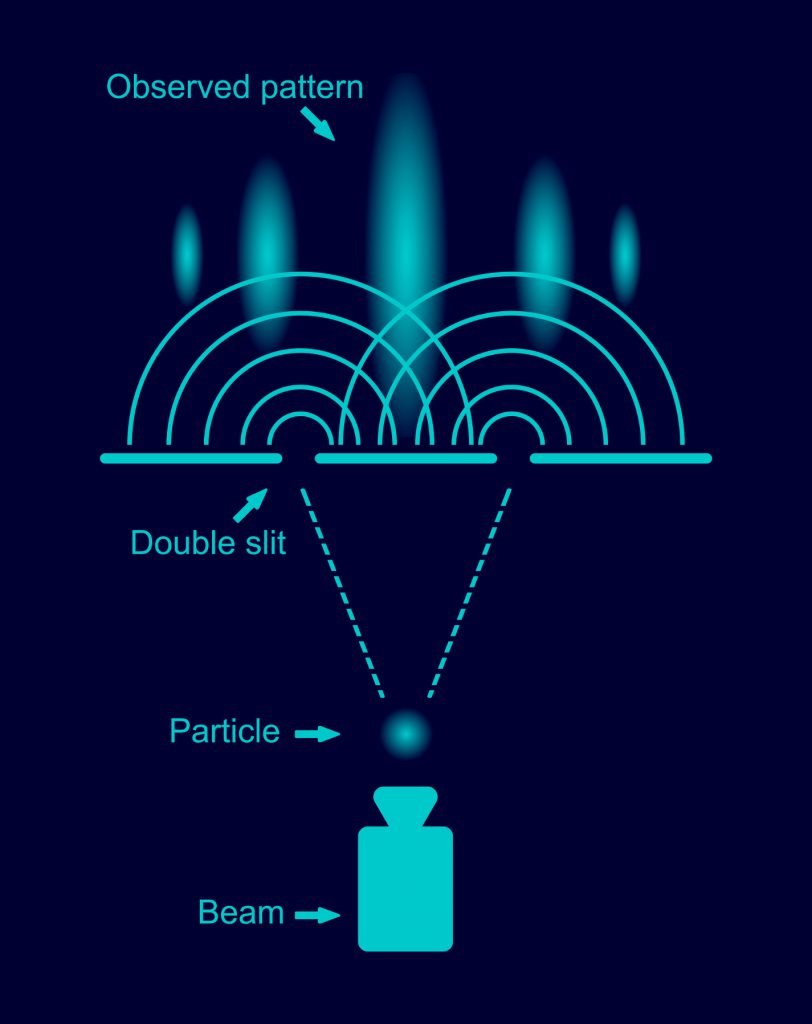
But let us first define what we mean by “classical mechanics.” It refers to physics before the 20th century. Back then, scientists thought that they were very close to a complete description of nature with everything being particles. Likewise, it refers to physics at low speeds (nowhere near light-speed). In 1802 (through Thomas Young’s double-slit experiment), it was discovered, though, that light acts like a wave rather than like individual particles. Light cast through slits or holes results in an interference pattern (see Figure 3.6).
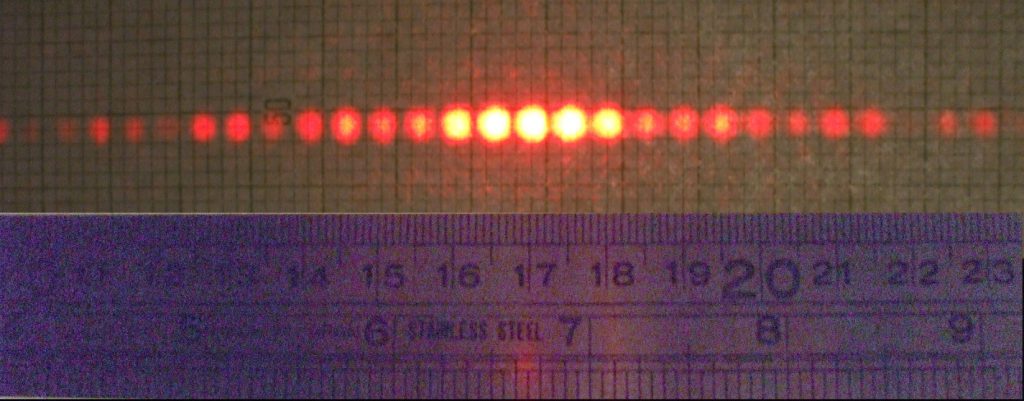
Example You can easily recreate the double-slit experiment with a laser pointer wrapped in aluminum foil. Use a needle to puncture one hole into the aluminum foil and compare the light pattern with the one after having punctured a second hole into the aluminum foil. You will see an interference pattern similar to that of water waves: if you throw two stones into the water, the hills and valleys of the waves overlap.
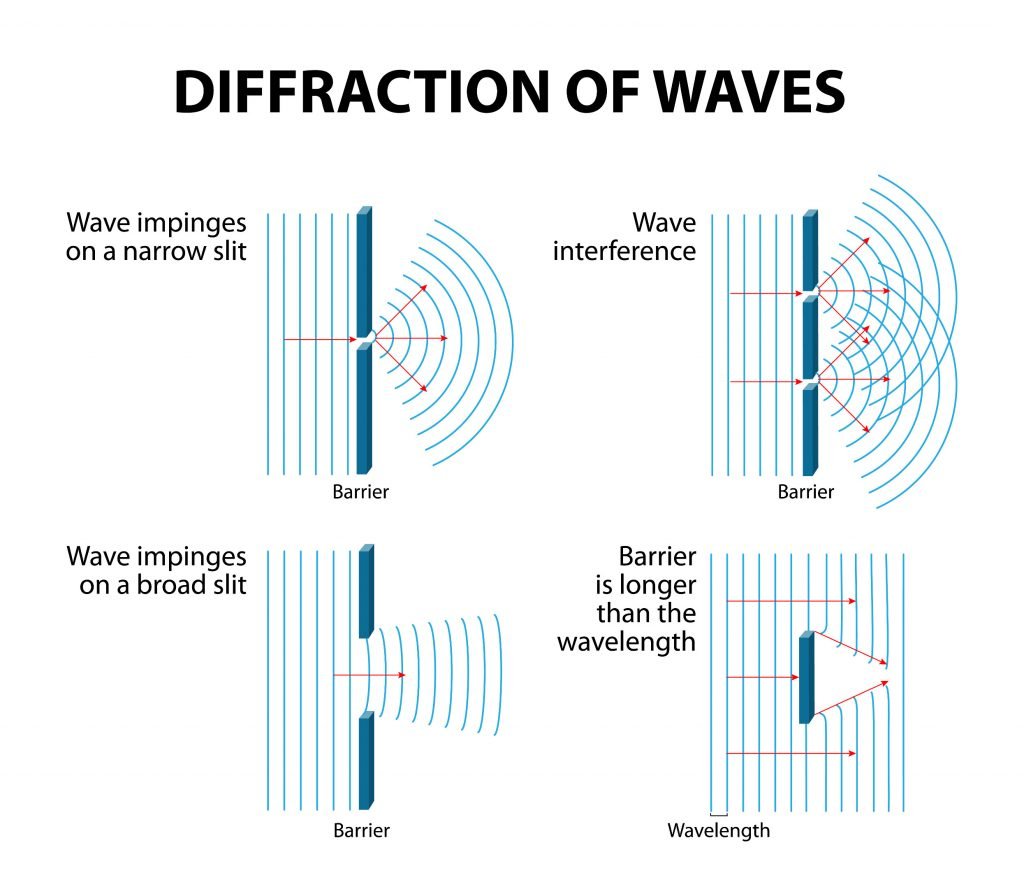
Generally, water waves are a good model for the interference pattern of light. On the following pages, you can see real world examples and schematics illustrating the properties of waves (see Figures 3.7, 3.8, and 3.9).

The idea that light is a wave was cast into question later when examining thermal radiation, the light that a heated object emits. Again, something you can observe yourself when heating up an oven and watching how the coils turn from black (infrared), to red, to white. If you had tools to objectively record the temperature and the wavelengths and intensity of the light, you would see how the intensity of the light first rises, and then sharply drops off for wavelengths below ultraviolet light (see Figure 3.10):
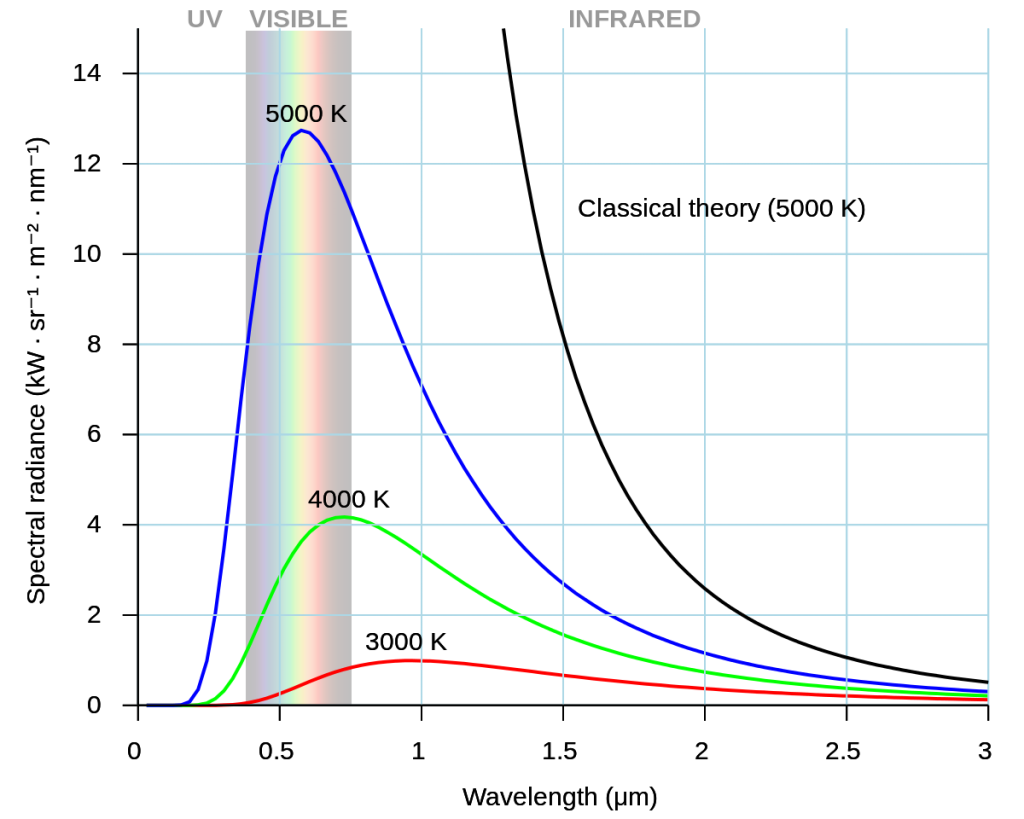
How did the scientists come up with a theory for thermal radiation using classical mechanics? The task was to calculate the amount of energy released by an object depending on the wavelength. The simple approach was to take a cube and calculate how many waves of a certain wavelength fit into it. This showed accurate results for larger wavelengths, but catastrophically failed for smaller wavelengths. Once the wavelength tends toward zero, the supposed emitted amount of energy by the object would tend toward infinity.
The solution was to go back to the original idea: light has properties of waves and particles. The idea was that light comes in packages (“quanta”—the plural of quantum which is Latin for “small amount”). Heat causes electrons of an atom to move to a higher level of energy. When the electrons return to their normal level, photons are generated and emitted as an electromagnetic wave.
But these different levels of energy are not continuous. Instead, you can imagine them like distinct electric neighborhoods around the atom. Either the energy is enough and they are pushed into the other neighborhood, or they do not move at all. The resulting photon has a fixed speed (light speed), no mass, and an energy dependent on its wavelength.
Example Think of a vending machine that accepts only quarters and not pennies: no matter how many pennies you put in, it will never add up to a quarter. Likewise, imagine an on/off switch on the machine that you cannot push continuously, but either it does not move at all, or it moves all the way into one or the other position. This is what the energy states of electrons are like.
Example The wavelength of a photon can be compared to sound: when you hit a drum, the membrane vibrates back and forth. This leads to the air being compressed and uncompressed in a regular frequency. This local oscillation is propagated throughout the room until it hits, for example, your eardrums, translating the oscillation of the air to a vibration of your membranes to nerve signals. Larger drums vibrate more slowly, leading to longer (deeper sounding) wavelengths. As opposed to moving air molecules, the photon vibrates the electric field. These vibrations can likewise cause cells in your eyes to be activated, translating them into nerve signals as well. Electromagnetic waves with larger wavelengths are called infrared, radar, microwave, or radio waves.
Light has properties of both waves and particles. It causes interference patterns like waves, but also comes in packages (“quanta”), hence the name “quantum theory” was born.
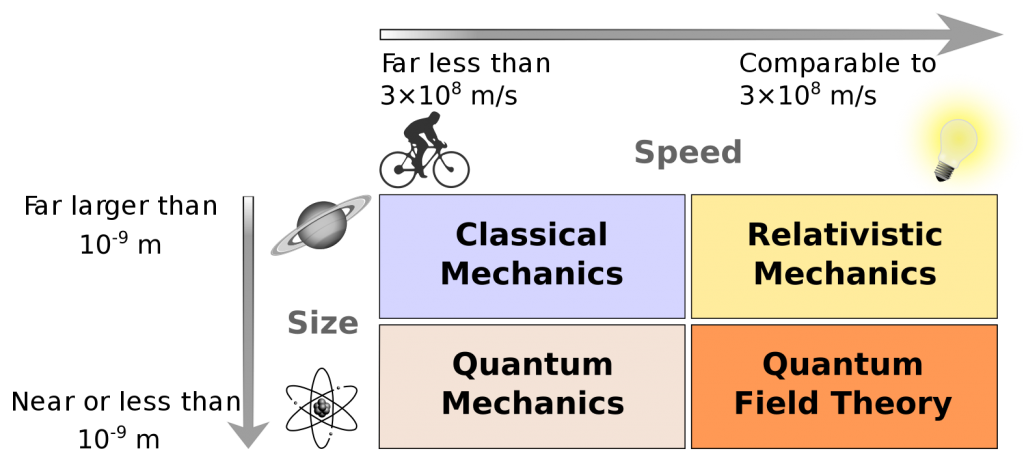
On top of quantum mechanics, relativistic mechanics was developed in 1905 with the publication of Einstein’s paper on special relativity (Zur Elektrodynamik bewegter Körper). Putting everything together, we can distinguish four major fields in physics distinguished by energy and speed (see Figure 3.11): classical mechanics (low speed, high energy), relativistic mechanics (high speed, high energy), quantum mechanics (low speed, low energy), and quantum field theory (high speed, low energy).
Quantum Computing
How can a quantum computer be significantly faster than a conventional digital computer?
A real-world application for the quantum theory is quantum computing. This field of science was initiated by the work of Paul Benioff and Yuri Manin in 1980, Richard Feynman in 1982, and David Deutsch in 1985. To understand quantum computing, let us first examine classical (digital) computing. Classical computing is based on binary digits (bits): either there is a current, or there is no current. With this basic distinction, logical “gates” work. Gates are small electrical relays with input and output wires.
Example In classical computers, the logical AND gate has two input wires and one output wire. When there is current on both input wires, the AND gate creates current on the output wire. If there is no current or current on only one of the wires, there is no output current. Similarly, OR, XOR, NXOR, NOT, etc. gates can be configured, with different input and output configurations. With these simple mechanisms, computers can be built.
QUBIT · A qubit can be compared to a wave (as opposed to a discrete unit as with digital bits). Combining several qubits with a quantum gate results in an instant calculation of an interference pattern between the waves, which can be used to significantly speed up processing.
Now, what about quantum computing? Quantum computers also have gates and “bits,” but both are different than the classical electrical bits and gates. Hence, they are called “quantum bits” (qubits) and “quantum gates.” To understand how those quantum gates work, we need to remember what we have said about quantum mechanics: quantum mechanics is basically wave mechanics. And a quantum computer is basically a “wave computer.” How can we build a “wave computer”? For that, let us go back to one of our examples: water waves and the double-slit experiment. Like the electrical AND gate, the double-slit setup has two inputs (the two slits) and one output (the screen). If we send no wave (or close both slits), the screen will show no pattern, the water is quiet. If we close either one of the slits, a single wave hits the screen. If we open both slits, we will see an interference pattern. So, already, we have some kind of “gate” here: an interference pattern will show up only if we send waves through both slits. There exists only “no wave” (0) or “wave” (1), and waves you send through the slits can be of any configuration between 0 and 1. Now, imagine a 10-slit setup: already, this configuration “calculates” the interference pattern of 1,024 possible different inputs! Depending on whether you are sending waves through individual slits or not, a different interference pattern emerges.
Currently, a number of companies and organizations are trying to build larger quantum computers. Depending on what counts as a quantum computer, the ranges are between 50 and 1,000 qubits. With so many qubits, this computer would be much, much faster than a classical computer. Again, not because it does more operations per second, but because a single operation instantly calculates the interference pattern of all qubits. And more qubits do not just increase the speed in a linear fashion. Doubling the number of qubits does not simply double the speed but leads to a multi-fold increase of speed. The larger the problem, the faster such a quantum computer would be compared to a classical computer.
The challenge of actually building such a quantum computer lies in quantum mechanics: in order for those quantum gates to work, they have to be cooled down to (near) absolute zero and any electromagnetic radiation needs to be shielded completely. Connecting all those qubits in a meaningful way and bridging the results into the “classical” world are additional challenges.
It might take another decade before true quantum computers become generally available, though, because error correction and reliability are still significant issues. Ultimately, a quantum computer ditches the idea of having a digital near-100% accurate result (the actual source of computation errors in digital computing is the memory, not the processor.) in favor of speed: it gives you the option if you can live with it—quick calculation with the possibility of false positives might sometimes be more important than 100% accuracy.
Quantum computing is so much more powerful than conventional computing because the way the waves interfere with each other is “computed” by nature for free. Basically, quantum computers repeatedly run small physics experiments, which makes solving problems of a similar nature very efficient. The difference, compared to a conventional computer, is that the interference pattern emerges in a few steps depending on the accuracy you want. Thus, the calculations of a quantum computer are much less problem-size dependent.
Summary
Light has properties of both waves and particles. It causes interference patterns like waves, but also comes in packages (“quanta”), hence the name “quantum theory” was born.
Quantum computing is so much more powerful than conventional computing because the way the waves interfere with each other is “computed” by nature for free. Basically, quantum computers repeatedly run small physics experiments, which makes solving problems of a similar nature very efficient. The difference, compared to a conventional computer, is that the interference pattern emerges in a few steps depending on the accuracy you want. Thus, the calculations of a quantum computer are much less problem-size dependent.












